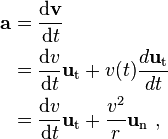Acceleration - Tangential and Centripetal Acceleration

Tangential and Centripetal Acceleration

See also: Local coordinates

The velocity of a particle moving on a curved path as a function of time can be written as:

with v(t) equal to the speed of travel along the path, and

a unit vector tangent to the path pointing in the direction of motion at the chosen moment in time. Taking into account both the changing speed v(t) and the changing direction of ut, the acceleration of a particle moving on a curved path on a planar surface can be written using the chain rule of differentiation and the derivative of the product of two functions of time as:

\begin{alignat}{3} \mathbf{a} & = \frac{\mathrm{d} \mathbf{v}}{\mathrm{d}t} \\ & = \frac{\mathrm{d}v }{\mathrm{d}t} \mathbf{u}_\mathrm{t} +v(t)\frac{d \mathbf{u}_\mathrm{t}}{dt} \\ & = \frac{\mathrm{d}v }{\mathrm{d}t} \mathbf{u}_\mathrm{t}+ \frac{v^2}{r}\mathbf{u}_\mathrm{n}\, \\ \end{alignat}

where un is the unit (inward) normal vector to the particle's trajectory, and r is its instantaneous radius of curvature based upon the osculating circle at time t. These components are called the tangential acceleration and the radial acceleration or centripetal acceleration (see also circular motion and centripetal force).

Extension of this approach to three-dimensional space curves that cannot be contained on a planar surface leads to the Frenet–Serret formulas.

Read more about this topic:  Acceleration

Famous quotes containing the words tangential and/or centripetal:

    “New York is full of people ... with a feeling for the tangential adventure, the risky adventure, the interlude that’s not likely to end in any double-ring ceremony.”
    —Joan Didion (b. 1934)

    “There is a relation between the hours of our life and the centuries of time. As the air I breathe is drawn from the great repositories of nature, as the light on my book is yielded by a star a hundred millions of miles distant, as the poise of my body depends on the equilibrium of centrifugal and centripetal forces, so the hours should be instructed by the ages and the ages explained by the hours.”
    —Ralph Waldo Emerson (1803–1882)