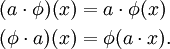Supermodule - Homomorphisms

Homomorphisms

A homomorphism between supermodules is a module homomorphism that preserves the grading. Let E and F be right supermodules over A. A map

is a supermodule homomorphism if

for all a∈A and all x,y∈E. The set of all module homomorphisms from E to F is denoted by Hom(E, F).

In many cases, it is necessary or convenient to consider a larger class of morphisms between supermodules. Let A be a supercommutative algebra. Then all supermodules over A be regarded as superbimodules in a natural fashion. For supermodules E and F, let Hom(E, F) denote the space of all right A-linear maps (i.e. all module homomorphisms from E to F considered as ungraded right A-modules). There is a natural grading on Hom(E, F) where the even homomorphisms are those that preserve the grading

and the odd homomorphisms are those that reverse the grading

If φ ∈ Hom(E, F) and a ∈ A are homogeneous then

That is, the even homomorphisms are both right and left linear whereas the odd homomorphism are right linear but left antilinear (with respect to the grading automorphism).

The set Hom(E, F) can be given the structure of a bimodule over A by setting

With the above grading Hom(E, F) becomes a supermodule over A whose even part is the set of all ordinary supermodule homomorphisms

In the language of category theory, the class of all supermodules over A forms a category with supermodule homomorphisms as the morphisms. This category is a symmetric monoidal closed category under the super tensor product whose internal Hom functor is given by Hom.