Multinomial Opinions
Let be a frame, i.e. a set of exhaustive and mutually disjoint propositions . A multinomial opinion over is the composite function, where is a vector of belief masses over the propositions of, is the uncertainty mass, and is a vector of base rate values over the propositions of . These components satisfy and as well as .
Visualising multinomial opinions is not trivial. Trinomial opinions could be visualised as points inside a triangular pyramid, but the 2D aspect of computer monitors would make this impractical. Opinions with dimensions larger than trinomial do not lend themselves to traditional visualisation.
Dirichlet distributions are normally denoted as where represents its parameters. The Dirichlet distribution of a multinomial opinion is the function 
where the vector components are given by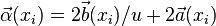 
Read more about this topic: Subjective Logic, Subjective Opinions
Famous quotes containing the word opinions:
“Loyalty to petrified opinions never yet broke a chain or freed a human soul in this world—and never will.”
—Mark Twain [Samuel Langhorne Clemens] (1835–1910)