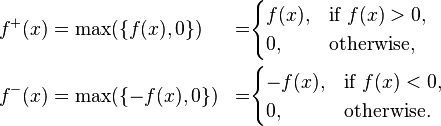Lebesgue Integral
It is often of interest, both in theory and applications, to be able to pass to the limit under the integral. For instance, a sequence of functions can frequently be constructed that approximate, in a suitable sense, the solution to a problem. Then the integral of the solution function should be the limit of the integrals of the approximations. However, many functions that can be obtained as limits are not Riemann integrable, and so such limit theorems do not hold with the Riemann integral. Therefore it is of great importance to have a definition of the integral that allows a wider class of functions to be integrated (Rudin 1987).
Such an integral is the Lebesgue integral, that exploits the following fact to enlarge the class of integrable functions: if the values of a function are rearranged over the domain, the integral of a function should remain the same. Thus Henri Lebesgue introduced the integral bearing his name, explaining this integral thus in a letter to Paul Montel:
I have to pay a certain sum, which I have collected in my pocket. I take the bills and coins out of my pocket and give them to the creditor in the order I find them until I have reached the total sum. This is the Riemann integral. But I can proceed differently. After I have taken all the money out of my pocket I order the bills and coins according to identical values and then I pay the several heaps one after the other to the creditor. This is my integral.As Folland (1984, p. 56) puts it, "To compute the Riemann integral of f, one partitions the domain into subintervals", while in the Lebesgue integral, "one is in effect partitioning the range of f". The definition of the Lebesgue integral thus begins with a measure, μ. In the simplest case, the Lebesgue measure μ(A) of an interval A = is its width, b − a, so that the Lebesgue integral agrees with the (proper) Riemann integral when both exist. In more complicated cases, the sets being measured can be highly fragmented, with no continuity and no resemblance to intervals.
Using the "partitioning the range of f" philosophy, the integral of a non-negative function f : R → R should be the sum over t of the areas between a thin horizontal strip between y = t and y = t + dt. This area is just μ{ x : f(x) > t} dt. Let f∗(t) = μ{ x : f(x) > t}. The Lebesgue integral of f is then defined by (Lieb & Loss 2001)
where the integral on the right is an ordinary improper Riemann integral (note that f∗ is a strictly decreasing positive function, and therefore has a well-defined improper Riemann integral). For a suitable class of functions (the measurable functions) this defines the Lebesgue integral.
A general measurable function f is Lebesgue integrable if the area between the graph of f and the x-axis is finite:
In that case, the integral is, as in the Riemannian case, the difference between the area above the x-axis and the area below the x-axis:
where
Read more about this topic: Integral, Formal Definitions
Famous quotes containing the word integral:
“Self-centeredness is a natural outgrowth of one of the toddler’s major concerns: What is me and what is mine...? This is why most toddlers are incapable of sharing ... to a toddler, what’s his is what he can get his hands on.... When something is taken away from him, he feels as though a piece of him—an integral piece—is being torn from him.”
—Lawrence Balter (20th century)