Annuity (finance Theory) - Annuity-due

An annuity-due is an annuity whose payments are made at the beginning of each period. Deposits in savings, rent or lease payments, and insurance premiums are examples of annuities due.


↓ ↓ ... ↓ payments
——— ——— ——— ——— —
0 1 ... n-1 n periods


Because each annuity payment is allowed to compound for one extra period. Thus, the present and future values of an annuity-due can be calculated through the formula:

\ddot{a}_{\overline{n|}i} = (1+i) \times a_{\overline{n|}i} = \frac{1-\left(1+i\right)^{-n}}{d}

and

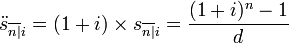
\ddot{s}_{\overline{n|}i} = (1+i) \times s_{\overline{n|}i} = \frac{(1+i)^n-1}{d}

where are the number of terms, is the per term interest rate, and is the effective rate of discount given by .


Future and present values for annuities due are related as:

\ddot{s}_{\overline{n}|i} = (1+i)^n \times \ddot{a}_{\overline{n}|i}

and

\frac{1}{\ddot{a}_{\overline{n}|i}} - \frac{1}{\ddot{s}_{\overline{n}|i}} = d

Example: The final value of a 7 year annuity-due with annual interest rate 9% and monthly payments of $100:

FV_{due}(0.09/12,7\times 12,$100) = $100 \times \ddot{a}_{\overline{84}|0.0075} = $11,730.01.

Note that in Excel, the PV and FV functions take on optional fifth argument which selects from annuity-immediate or annuity-due.

An annuity-due with n payments is the sum of one annuity payment now and an ordinary annuity with one payment less, and also equal, with a time shift, to an ordinary annuity with one payment more, minus the last payment. Thus we have:


(value at the time of the first of n payments of 1)
(value one period after the time of the last of n payments of 1)

Read more about this topic:  Annuity (finance Theory)