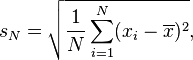Uncorrected Sample Standard Deviation
Firstly, the formula for the population standard deviation (of a finite population) can be applied to the sample, using the size of the sample as the size of the population (though the actual population size from which the sample is drawn may be much larger). This estimator, denoted by sN, is known as the uncorrected sample standard deviation, or sometimes the standard deviation of the sample (considered as the entire population), and is defined as follows:
where are the observed values of the sample items and is the mean value of these observations, while the denominator N stands for the size of the sample.
This is a consistent estimator (it converges in probability to the population value as the number of samples goes to infinity), and is the maximum-likelihood estimate when the population is normally distributed. However, this is a biased estimator, as the estimates are generally too low. The bias decreases as sample size grows, dropping off as 1/n, and thus is most significant for small or moderate sample sizes; for the bias is below 1%. Thus for very large sample sizes, the uncorrected sample standard deviation is generally acceptable. This estimator also has a uniformly smaller mean squared error than the corrected sample standard deviation.
Read more about this topic: Sample Standard Deviation, Estimation
Famous quotes containing the words uncorrected, sample and/or standard:
“Such uncorrected visions end in church
Or registrar:
A mortgaged semi- with a silver birch;
Nippers; the widowed mum; having to scheme
With money; illness; age.”
—Philip Larkin (1922–1986)
“The present war having so long cut off all communication with Great-Britain, we are not able to make a fair estimate of the state of science in that country. The spirit in which she wages war is the only sample before our eyes, and that does not seem the legitimate offspring either of science or of civilization.”
—Thomas Jefferson (1743–1826)
“Neither I nor anyone else knows what a standard is. We all recognize a dishonorable act, but have no idea what honor is.”
—Anton Pavlovich Chekhov (1860–1904)